Using time value of money for real estate valuation

How the time value of money applies to Real Estate Valuation - (It's Rocket Science)

When it comes to real estate, there are a lot of factors that go into the valuation process. Understanding the time value of money is among one of them. This principle states that money available at present is worth more than the same sum in the future due to its earning potential. 

Often, students and analysts learn about more complicated ways to value things like the internal rate of return (IRR) and net present value (NPV) in a way that doesn't make sense to them. The time value of money (TVM) is the fundamental concept that underlies the IRR and NPV approaches.

This article will walk you through the important concept of the time value of money, and then you will find some examples of why it's so crucial to understand the impact of the timing of cash flows on a real estate investment.

What is the time value of money in real estate?

Time value of money definition:

It is the idea that one dollar today is worth more than one dollar tomorrow. The dollar today can be invested and earn interest, while the dollar tomorrow cannot.

This principle depends on compound interest, which states that earnings on an investment will grow at a compounded rate over time. 

The earnings from the initial investments will be reinvested and then earn interest on top of that. So, the value of money grows over time as long as it is invested in a compounding interest account. 

Let's understand the concept of the time value of money with an example -  

Assume a $250 investor is weighing three 10-year investment options. These are the alternatives:

• Plan A: The investor gets $20 after the first year and the original $250 after ten years.
• Plan B: The investor gets $2 every year for ten years, plus the original $250.
• Plan C: After the tenth year, the investor will receive $20 plus the original $250.

Each plan pays out $250 plus $20 every year for ten years.

Which strategy should the investor choose?

Plan A is the best option for the investor. Why? Because when money is received, it can be allocated to one of two purposes. 

The investor could use the funds to treat a friend to dinner. Second, the money can be invested by the investor. 

By spending or reinvesting the $10 acquired at the end of year 1, the investor has the greatest possibility to spend the money or earn interest throughout the 10-year term with Plan A. 

Plan A allows him to invest ten dollars for nine years and earn more interest than Plan B allows for ten one-year investments of $1 each. Because of the possibility to collect interest, investors who plan to reinvest their returns prefer earlier returns over later ones. 

Early returns are preferred by real estate investors who consume their gains simultaneously. Would you want to wait ten years to take your friend out to dinner, as Plan C requires?

Timeline in time value of money

Panel A of the below figure depicts a timeline, which serves as visual assistance for visualising the time pattern of money returns. 

Beginning on the left with the present, time 0, and ending on the right with the terminal time point, N, the line is broken into time points and time intervals, or periods.

When reviewing single-property investments, annual periods are commonly utilised. However, monthly periods are sometimes suitable (for example, when analysing mortgage or lease payments).

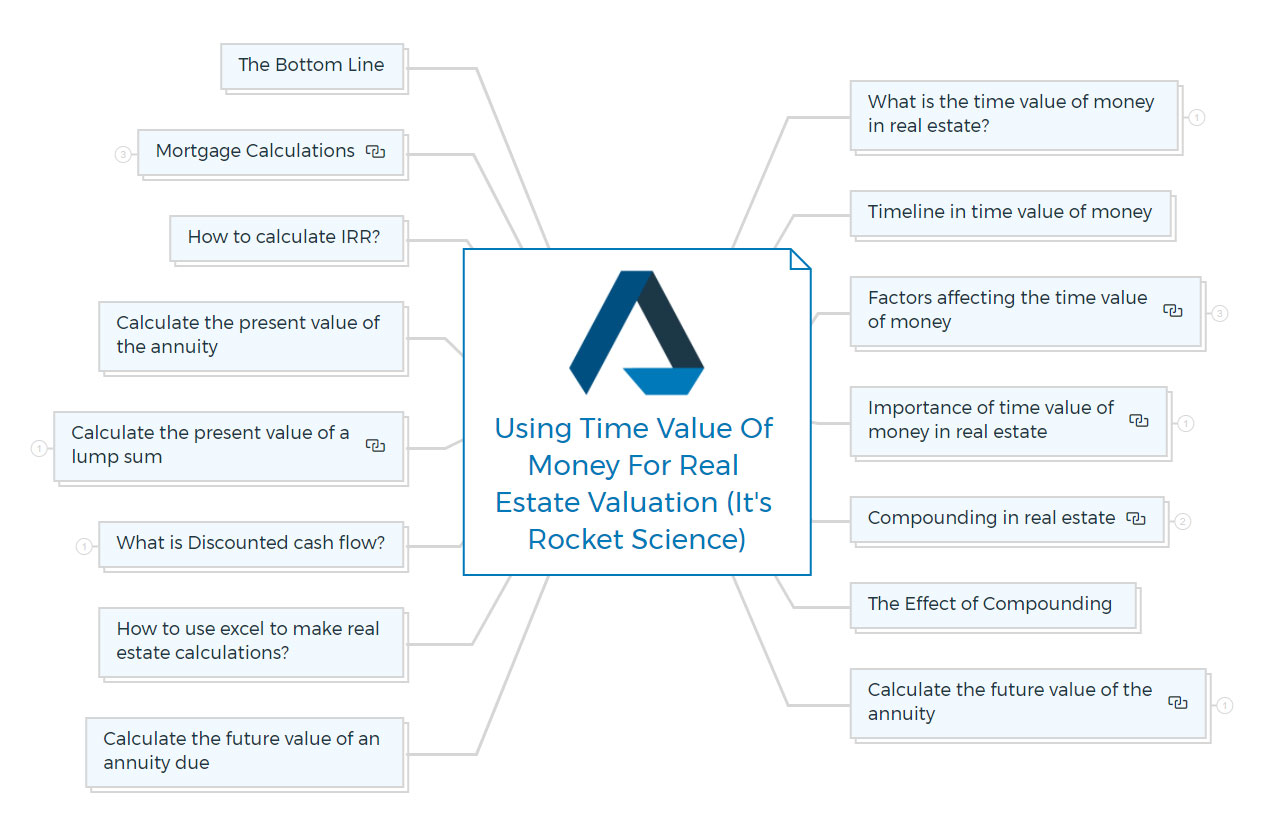
Factors affecting the time value of money

A few factors will affect the time value of money in a real estate context. These include:

The interest rate

The higher the interest rate, the greater the impact of compound interest on the value of money.

The amount of time

The longer the period, the greater the impact of compound interest.

The starting point

The earlier the cash flow begins, the greater the impact of compound interest.

Importance of time value of money in real estate

The time value of money is one of the most important concepts in real estate investment.

• It considers that cash flows from a real estate investment are not always received simultaneously. 

When valuing a property, it's important to discount future cash flows back to their present value. You can do this by using an appropriate discount rate, the interest rate you would need to earn to have the same buying power as the cash flow in question. 

This discount rate is based on the time value of money and considers both the amount of time and the uncertainty of whether or not it will occur. 

When you're trying to decide whether or not to invest in a property, it's important to look at the investment's net present value (NPV). The NPV is calculated by summing up all the cash flows associated with the investment and then discounting them to their present value. 

It considers both the positive and negative cash flows and measures whether or not the investment is worth making.

• The time value of money is also important for refinancing a property.

You exchange one set of future cash flows for another when you refinance. It's important to ensure that the new cash flows are worth more than the old ones; otherwise, you're not gaining anything by refinancing.

• Finally, the time value of money is important for selling a property.

When you sell a commercial property, you exchange all future cash flows for a lump sum of cash from the investment. It's important to ensure that the lump sum is worth more than the future cash flows; otherwise, you're not gaining anything by selling.

In short, the time value of money is a very important concept in real estate investment. You can better understand the basic financial concept of real estate deals and make more informed investment decisions by understanding them.

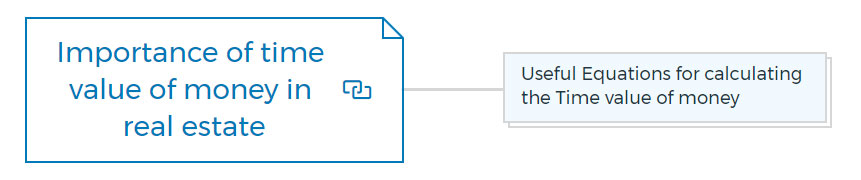
Equations for calculating the time value of money

Compounding in real estate

The first two time-value-of-money (TVM) procedures are utilised to determine future values resulting from compounding interest. Compound interest is when an investor earns interest on the principal and the accrued interest.

Calculate the future value of a lump sum

Assume $1,000 is invested in an investment account managed by a financial advisor today.

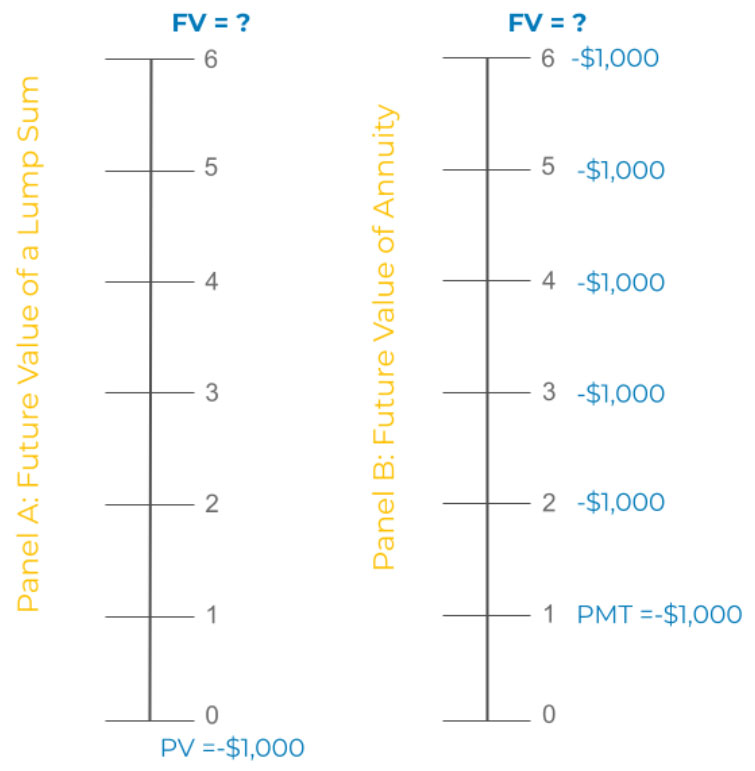
The investor plans to take the original investment plus accrued earnings at the end of 6 years, and the account is predicted to produce a 7.5 % annual compounded return. 

Panel A shows the cash inflows and outflows in a timeline format for this situation. 

The present value (PV) of $1,000 invested at time zero is transformed to a future value (FV) at the end of 6 years to solve this problem. 

The PV is multiplied by the future value interest factor (FVF) for the selected interest rate and the time to make the conversion. The calculation for converting a present value into a future value is -

Future value of lump sum formula

FV = PV X FVF (7.5 percent, six years)

= $1000 X 1.54

= $1,543.30

The solution to the future value of a lump sum equation is the FVF of 1.54, assuming that $1 is invested at 7.5% for 6 years.  

It shows that $1 invested now at 7.5% for six years will grow (compound) to $1.54 at the end of 6 years (assuming annual compounding). As a result, if you invest $1,000 today, it will rise to $1543.30 in six years.  

To solve this problem, you'll need to use the following keystrokes on your calculator:

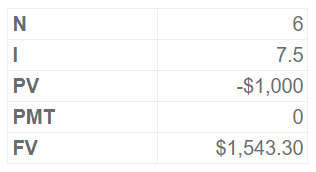
The $1,000 lump sum investment is entered as a negative figure, indicating an outflow (i.e., cash paid) from the investor's perspective. 

Entering N, I, PV, and PMT (the "knowns") into a calculator and pressing the FV key (the "unknown") yields the $1543.30 solution. 

With a 12 percent interest rate, $1,000 invested now will increase to $1,973.82 in six years. The calculator keystrokes and conversion equation are listed below.

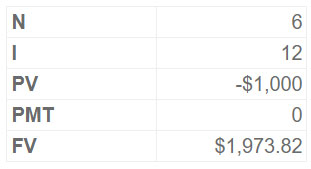
FV = PV X FVF (12%, six years)

= $1,000 x 1.97

= $1,973.82

It's worth noting that you can figure out the fifth if you know four of the five essential inputs. 

Consider the case where the future value of $1,973.82 is known, but I, the yearly interest rate, is unknown. You can obtain a solution of 12% by entering $1,973.82 in the FV register (along with N = 6, PV = -$1,000, and PMT = 0) and pressing the I key.

The effect of compounding

We explore the growth rate in the $1,000 investment above in greater detail to demonstrate the power of compounding. 

The below table depicts how much the lump sum investment will grow, assuming interest is compounded (paid) annually and the investor does not remove any of the $1,000 initial investment or interest earnings.

The value of the investment remains constant throughout the year because of annual compounding. The accumulated investment amount rises by the interest generated during the first year at the end of the year. 

If the investor withdraws the money after a year, the total value of the investment will be $1,120, which includes the $1,000 initial investment plus $120 in interest income. 

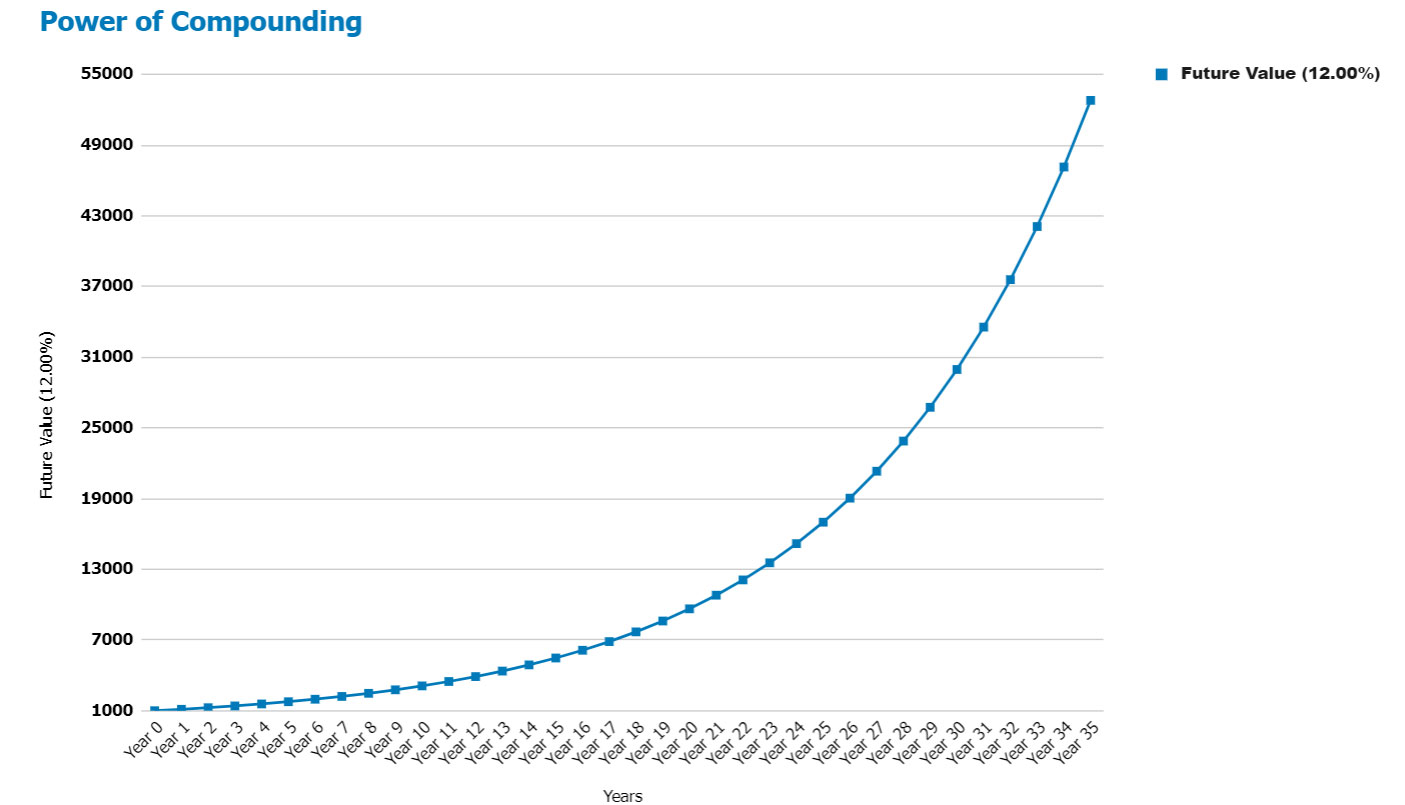
After seven years, the aggregate investment value will be $2,211, equal to the $1,000 investment plus $1,211 in interest income. By the completion of the 35th year, the investment will be worth $52,799. 

It's worth noting that the average amount of interest earned goes up as the length of time goes on. It is because interest is being earned on a larger and larger investment. 

As a result, the longer an investment is allowed to compound, the more powerful compounding becomes!

The below graph shows the growth of a $1,000 investment over time using annual compounding at 12%. It's worth noting that the slope of the curve rises as the investment holding term lengthens.

Interest is compounded annually in this example. On the other hand, banks and other borrowers frequently pay interest monthly or even daily. 

The table below compares the accumulated investment values for a $1,000 investment assuming monthly and daily compounding to the accumulated investment values assuming annual compounding.

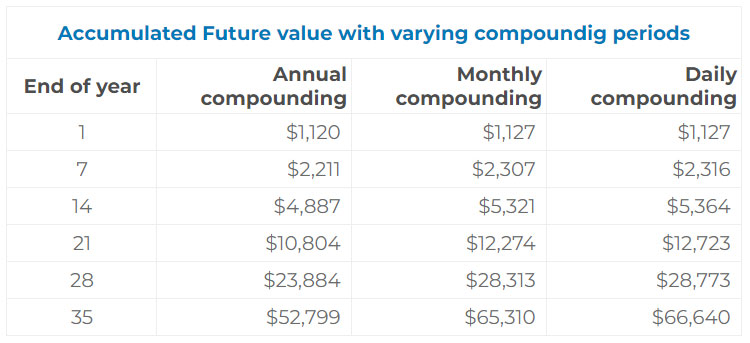
With monthly compounding, a $1,000 investment will grow to $2,307 over seven years, compared to $2,211 with yearly compounding. The investment will have grown to $2,316 in seven years due to the daily compounding of interest. 

As the investing time horizon grows longer, the impacts of more frequent compounding become more obvious. We'll presume that compounding frequency is the same as the frequency with which the payments are made or received unless otherwise indicated. 

If cash flows are received yearly, we shall assume annual compounding.

Calculate the future value of the annuity

Assume the investor intends to invest $1,000 in a 7.5% yearly compounded investment at the end of each year and wants to know how much these six investments will be worth. 

Solving this problem entails determining the future value of an annuity, as indicated in panel B of the figure of timeline demonstration for compounding problems. 

The $1,000 annuity (i.e., cash investments at the end of each period) is converted into future values using future value factors for annuities (FVFA).

Future value of annuity formula

FVA = Annuity X FVFA (7.5%, six years)
= $1000 X 7.24
= $7,244.02

The FVFA of 7.24402 is the answer to the future value of an annuity equation at 7.5% for six years. It shows that investing $1 at the end of each year for six years at 7.5% yields $7.24402 at the end of the six years. 

As a result, a $1,000 investment over six years will rise to $7,244.02. The keystrokes on the calculator are

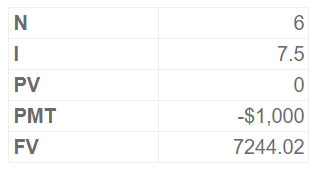
The future value is, once again, unknown. The level payment is entered as a negative number in the PMT register. The PV register includes a zero amount (or is empty) for calculating the future value of a series of level payments (investments). 

This instructs the calculator to invest the money every year for N years. Remember that the one-time investment is entered in the PV register when calculating the future value of a lump sum—the PMT register contains a zero or is empty. 

Finally, suppose the investor wants to make monthly investments of $83.33 ($1,000 12) over the next six years. The monthly interest rate, I, is 0.625 per cent (7.5 percent/12) in this case, and N is 72 (6X12).

FVA = Annuity X FVFA (7.5%/12, 72 months)
= $83.33 X $90.58
= $7,547.93

The relevant keystrokes are

Regardless of whether payments are made monthly or annually, the investor will invest $1,000 every year in this example.  

If monthly payments of $83.33 are made, however, after six years, the investor will have $7,547.93, compared to $7,244.02 with annual investments. 

Why is there a $303.9 difference in future values when both methods have the same total payments throughout the six years? In the monthly compounding scenario, the initial investment occurs at the end of month 1, whereas in the yearly compounding scenario, it occurs at the end of year 1.  

As a result, monthly investments begin to earn interest 11 months earlier than annual installments.

Calculate the future value of an annuity due

What if the investor wants to invest $1,000 every year for the next six years? These situations are the " future value of an annuity due." problems.

The total interest received over the five years will increase since the initial and subsequent $1,000 annual payments are brought forward a year. 

Users of financial calculators can easily specify that cash flows should be invested or received at the start of each period ("begin mode") rather than at the end ("end mode"). Other than that, the keystrokes are the same. 

The future value of this annuity due is $7,787.32, which is $543.3 more than the $7,244.02 accumulated value assuming year-end deposits. What is the link between the annuity due result and the predicted future value result? 

Note that you can get the $7,787.32 annuity due solution by multiplying the standard annuity problem solution by 1 plus the monthly interest rate [i.e. $7,787.32 = $7244.02 (1 + 0.075)]. 

Assume that a 12-unit apartment building owner will put $1,200 in an interest-bearing reserve account each year, or $100 per unit. After seven years, the proceeds will be utilised to recondition the units.  

What will the total value of the reserve account be at the end of seven years if the deposits are made at the beginning of each year and earn 7.5% interest compounded annually?

How to use excel to make real estate calculations?

Excel has many financial features that can aid you with any real estate calculation. You can use one of them here.

Let's start.

Step 1

Begin by creating a new Excel worksheet.

Step 2

Select "Function" from the "Insert" menu that appears when you pull down the "Insert" menu. You'll see something like this in the window:

Step 3

You'll find function categories in the left-hand pane. Choose "Financial."

And then, select NPER; it is a financial function in Excel that you may use to calculate the number of periods in various investment scenarios. 

Click "OK" after selecting that function in the right-hand window.

Step 4

Next, you'll see a window where you can enter values for each variable that this function might utilise. 

For our example, not all of the variables are required. Because you haven't made any payments, this can be overlooked.

The "type" specifies whether payments are made at the start or end of each period; the function defaults to the most common option so that you can disregard it as well. You must fill in the following information:

1. The "Rate" is .04 (the decimal equivalent of 4 per cent)
2. The "PV" as -200000 (note that you must express the PV as a negative number since PV is the money going out and FV is money coming in from the function's flow-of-funds investment perspective; also note that you do not use commas within the numbers)
3. The FV is set to 400000.

Step 5

You'll notice the formula result, 17.67299, at the bottom of the window. It means that at a 4% annual growth rate, it will take 17.67 years for the value of this property to increase from $200,000 to $400,000.

When you click the "OK" button, the window closes, and the result shows in the cell where your pointer was when you started the exercise. That cell now has a formula in it, which was generated by the form you filled out:

= NPER(0.04,, -200000,400000) 

You can skip the form and write a formula like this right into the cell if you become an Excel "superuser" in the future. You can calculate numerous financial functions using a form like the one above.

What is discounted cash flow?

The discounted cash flow calculation considers the time value of money by discounting future cash flows back to the present. DCF analysis uses a discount rate that reflects the risks associated with the cash flows and the expected time."

When valuing a property, real estate professionals often use discounted cash flow (DCF) technique.

How to calculate discounted cash flow (DCF)?

You can calculate DCF in three steps:

Step 1

Forecast the expected cash flows from the investment.

Step 2

You choose a discount rate, which depends on the cost of funding the investment or the opportunity cost of other investments.

Step 3

Using a financial calculator, a spreadsheet, or a manual computation, discount the predicted cash flows back to the present day.

Calculate the present value of a lump sum

The present value of future lump sum (i.e. one-time) receipts is calculated using this technique. Assume the investor has been provided with an investment opportunity to generate a $1,543.30 cash inflow over the next six years, as indicated in panel A below.

Assume that the investor expects to earn 7.5% on identical investments, compounded annually. The 7.5% discount rate can be considered an opportunity cost—the return that an investor foregoes on a risk-free alternative investment to engage in the current opportunity. 

How much can the investor pay today for this $1,543.30 future lump sum payment and make a 7.5% profit? 

To solve this problem, you need to convert a future lump sum amount receipt into a present value.

Present value of lump sum formula

PV = FV X PVF (7.5%, six years)
= $1543.30 X 0.65
= $1,000.00

If they can get a 7.5% annual return on similar assets, the investor should be willing to spend up to $1,000 today to receive $1543.30 at the end of six years. 

The keystrokes for the calculator are as follows:

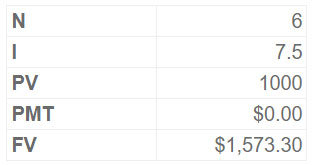
With these values, your calculator should return -$1,000. Because calculators require the matching of inflows (+) and outflows (-) to do TVM calculations, present values are displayed as negative amounts (assuming the future value is provided as a positive number).

You can use the discounting cash flow calculation to help you assess the profitability of a potential investment. 

Here are the top property investment strategies that you should know. 

Calculate the present value of the annuity

Assume that investors are presented with an investment opportunity to receive $1,543.30 at the end of each year for the next six years, as illustrated in panel B of the timeline presentation for discounting problems. 

Property investors that need a 7.5 percent yearly return should know how much they may spend for this annuity and still get the 7.5 percent return they need.  

There are two methods for translating an annuity into a present value. One method calculates the present value of six lump-sum amounts for each year and then adds the figures together. 

Calculating the present value of an annuity is a less time-consuming option (PVA). This alternate operation's conversion equation is

PVA = Annuity X PVFA (7.5%, six years)
= $1,543.30 X 4.694
= $7,244.01

Keystrokes required for this calculation are -

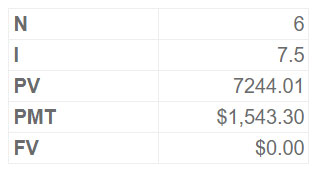
The annuity amount is placed into the PMT register because the cash flows will be received every year for the next six years. There is no fixed quantity (FV). 

What if the payments are due at the start of each calendar year? The present value of this annuity is $7,787.31, which may be computed using the "begin mode" on your calculator or by increasing the present value of the standard annuity by an additional year's worth of interest.

 [i.e., $7,787.31 = $7,244.01 (1 + 0.075)].

How to calculate IRR?

Now we know,

1. How to calculate future values when the amount to be invested is known (compounding) and
2. How to arrive at current values while future values are known or assumed (i.e., discounting).

You can use the TVM concept to calculate real estate investment rates of return, which is a critical extension.

Consider the future value of a lump sum problem we looked at earlier. We found that if $1,000 is deposited today for six years in an investment vehicle that earns 7.5% compounded annually, the account amount at the end of five years will be $1,543.30.

As a result, making this investment is equivalent to earning a 75% return. The investment yield or internal rate of return (IRR) are terms used to describe this rate of return.

Consider the present value of an annuity problem in which investors were offered the chance to receive $1,543.30 at the end of each year for the next six years. The current value of future cash flows is $7,244.01 if investors believe they can get a 7.5% return on equivalent risk assets. 

What kind of return or IRR can investors expect if they invest $7,244.01 in this opportunity? Obviously, on their $7,244.01 investment, investors will get a 7.5% internal rate of return. The keystrokes on the calculator are

The above solution process will not function if the periodic cash flows from owning and renting real estate assets are not projected to remain consistent over time. Instead, investors must use spreadsheet software or their financial calculators' cash flow key. 

The internal rate of return (IRR) is crucial in real estate investment and finance. It expresses the return on investment throughout the entire investment holding term as a compound interest rate. 

On similar projects of comparable risk, it is easy to compare the computed (or "going-in") IRR to the investor's needed IRR. If an investment opportunity's going-in IRR exceeds the investor's necessary rate of return, the venture should be pursued. If the IRR at the outset is less than the investor's necessary rate of return, avoid the investment and explore other options.

Mortgage calculations

Understanding mortgage calculations is critical when purchasing a property. To make an informed decision, you need to know how much your monthly payment will be, how much money you will need for a down payment and the loan terms.  

Fortunately, several online calculators can help you with these calculations.

One of the most important mortgage calculations is the monthly payment. 

This calculation tells you how much money you need to pay each month to repay your loan over 30 years.  

To calculate your monthly payment, you need to know three things: 

• the amount of the loan
• the interest rate
• And the number of months in the loan.

Monthly payment calculation formula

PMT = (L x i) / [(r x n) – (l x i)]

Monthly payment calculation example

Suppose you have a 30-year mortgage with an interest rate of 12%. To calculate your monthly payment, you would use the following formula:

PMT = (L x i) / [(r x n) – (l x i)]

PMT = (30,000 x .12) / [(.12 x 360) – (30,000 x .12)]
PMT = $405.53

This means that your monthly payment would be $405.53.

To purchase a property, you will also need to know how much money you will need for a down payment.

Down payments can range from 0% to 20% of the property's purchase price. To calculate your required down payment, you need to know the purchase price of the property and the loan-to-value ratio.

Down Payment = (Purchase Price – Loan Amount) / (Loan Amount x 100)

Down payment calculation example

Suppose you want to purchase a property for $200,000. To calculate your required down payment, you would use the following equation:

Down Payment = (200,000 – 100,000) / (100,000 x 100)
Down Payment = $20,000

You will need to provide a down payment of $20,000 to purchase the property. 

Finally, it is important to understand the loan terms before you sign on the dotted line. The most common term for a mortgage is 30 years. However, you can also choose a 15-year or 20-year mortgage.

The Bottom line

As you can see, the time value of money is a critical concept in real estate valuation methods. By understanding how to calculate present and future values, you can make better decisions about your investments.  

In addition, by using Excel to perform these calculations or real estate valuation methods, you can become more comfortable with the numbers and better understand what goes into making sound real estate investment decisions.

Enroll to one of the structured property development courses to make your next project a success.